Awarded as the best online publication by CIDC

Design Calculation for Slab

Home Design Sheets

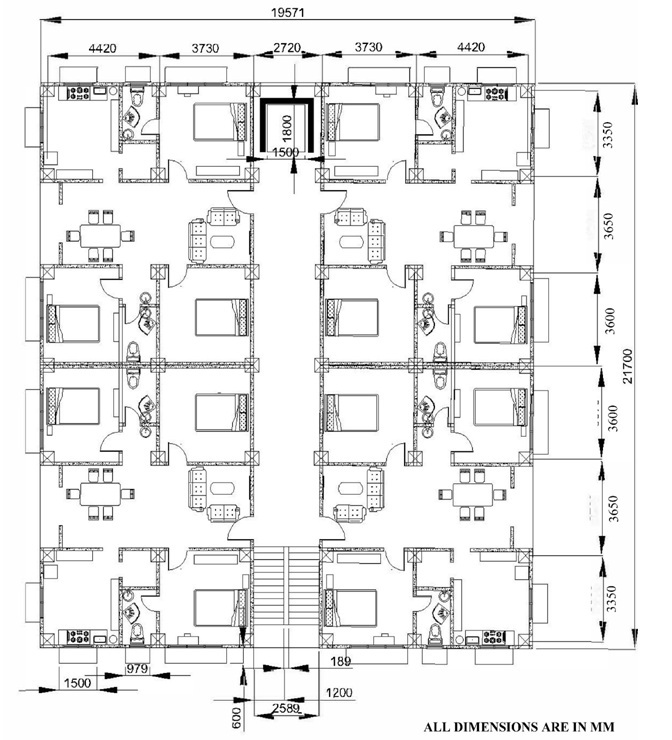
INTRODUCTION
A slab is a structural element that is used to support ceilings and floors. It is made of concrete and reinforcements are provided to support it. It is several inches thick and it rests on beams and columns. It is estimated that a concrete slab lasts for about 30 to 100 years if it is built with good grade of concrete and steel. Usually for residential purpose M20 is used.

There are two types of slabs – one-way slab and two-way slab. Two-way slabs are supported on four sides while one-way slabs are supported on two opposite sides. Two-way slabs carry load in two directions and so reinforcements are provided in both directions. Whereas in one-way slab the reinforcement is provided in one direction as it carries load in one direction. In one way slab the ratio of longer to smaller span is greater than or equal to 2 while in two-way slab the ratio of longer to smaller span is less than 2.

Here, in this problem first a plan of the house is shown then the slab panels are depicted as per the house plan. The plan used for gravity analysis of a building is similar to slab panels also. Here primary beams are taken and secondary beams are not included in order to make the calculations short. Also, the longer to shorter span ratio is less than 2 in all panel slabs so all slabs panels are two-way slabs. The design method is followed as per Annexure D of IS 456:2000.

Building Plan Dimensions

Slab Panel

DESIGN OF SLAB:
Design of typical Floor Slab:
We have thickness of slab as 130 mm.
Considering M20 grade of concrete and Fe 500 steel of diameter of 10 mm
Effective Depth: (130-15-10/2) = 110 mm.

Load Calculation:
Live Load = 2 kN/m2
Self-weight of the slab = 3.25 kN/m2
Floor Finish = 1 kN/m2
Total = 6.25 kN/m2

Determination of Moments of slabs (Laterally Restrained slab):
According to IS 456:2000 Clause D-1.1, maximum bending moment per unit width in the slab is given by-
Mx = αxWlx2
My = αyWly2

Where lx and ly are the length of the shorter and longer span respectively,
αx, αyare moment coefficients,
Mx and My are moments on strips of unit width spanning lx and ly respectively,
W= total design load per unit area.

Check for Thickness
Check for Thickness

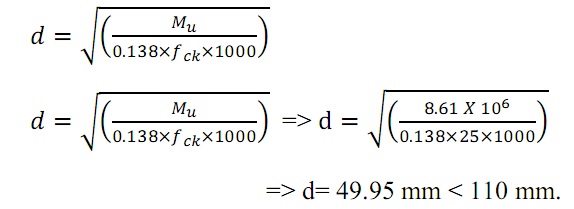
Here the highest moment is taken from the table below.
Hence Safe

Calculation of B.M. Coefficient

Panel no. lx (m) ly (m) ly/lx Type Negative αx Negative αy Positive αx Positive αy
1 3.35 4.42 1.32 Two adjacent edges discontinuous 0.066 0.047 0.050 0.035
2 3.35 3.73 1.11 One long edge discontinuous 0.045 0.037 0.034 0.028
3 3.35 3.73 1.11 One long edge discontinuous 0.045 0.037 0.034 0.028
4 3.35 4.42 1.32 Two adjacent edges discontinuous 0.066 0.047 0.050 0.035
5 3.65 4.42 1.21 One short edge discontinuous 0.048 0.037 0.036 0.028
6 3.65 3.73 1.02 Interior panel 0.033 0.032 0.025 0.024
7 2.72 3.65 1.34 Interior panel 0.049 0.032 0.037 0.024
8 3.65 3.73 1.02 Interior panel 0.033 0.032 0.025 0.024
9 3.65 4.42 1.21 One short edge discontinuous 0.048 0.037 0.036 0.028
10 3.6 4.42 1.23 One short edge discontinuous 0.049 0.037 0.037 0.028
11 3.6 3.73 1.04 Interior panel 0.034 0.032 0.025 0.024
12 2.72 3.6 1.32 Interior panel 0.048 0.032 0.037 0.024
13 3.6 3.73 1.04 Interior panel 0.034 0.032 0.025 0.024
14 3.6 4.42 1.23 One short edge discontinuous 0.049 0.037 0.037 0.028
15 3.6 4.42 1.23 One short edge discontinuous 0.049 0.037 0.037 0.028
16 3.6 3.73 1.04 Interior panel 0.034 0.032 0.025 0.024
17 2.72 3.6 1.32 Interior panel 0.048 0.032 0.037 0.024
18 3.6 3.73 1.04 Interior panel 0.034 0.032 0.025 0.024
19 3.6 4.42 1.23 One short edge discontinuous 0.049 0.037 0.037 0.028
20 3.65 4.42 1.21 One short edge discontinuous 0.048 0.037 0.036 0.028
21 3.65 3.73 1.02 Interior panel 0.033 0.032 0.025 0.024
22 2.72 3.65 1.34 Interior panel 0.049 0.032 0.037 0.024
23 3.65 3.73 1.02 Interior panel 0.033 0.032 0.025 0.024
24 3.65 4.42 1.21 One short edge discontinuous 0.048 0.037 0.036 0.028
25 3.35 4.42 1.32 Two adjacent edges discontinuous 0.066 0.047 0.050 0.035
26 3.35 3.73 1.11 One long edge discontinuous 0.045 0.037 0.034 0.028
27 3.35 3.73 1.11 One long edge discontinuous 0.045 0.037 0.034 0.028
28 3.35 4.42 1.32 Two adjacent edges discontinuous 0.066 0.047 0.050 0.035

Calculations of Moments

Panel No Factored B.M for shorter direction Mx=αx wlx2 x 1.5 Factored B.M for longer direction My=αy wly2 x 1.5
Near Support (-ve) Near support (+ve) Near Support (-ve) Near support (+ve)
1 6.94 5.26 8.61 6.41
2 4.73 3.58 4.83 3.65
3 4.73 3.58 4.83 3.65
4 6.94 5.26 8.61 6.41
5 6.00 4.50 6.78 5.13
6 4.12 3.12 4.17 3.13
7 3.40 2.57 4.00 3.00
8 4.12 3.12 4.17 3.13
9 6.00 4.50 6.78 5.13
10 5.95 4.50 6.78 5.13
11 4.13 3.04 4.17 3.13
12 3.33 2.57 3.89 2.92
13 4.13 3.04 4.17 3.13
14 5.95 4.50 6.78 5.13
15 5.95 4.50 6.78 5.13
16 4.13 3.04 4.17 3.13
17 3.33 2.57 3.89 2.92
18 4.13 3.04 4.17 3.13
19 5.95 4.50 6.78 5.13
20 6.00 4.50 6.78 5.13
21 4.12 3.12 4.17 3.13
22 3.40 2.57 4.00 3.00
23 4.12 3.12 4.17 3.13
24 6.00 4.50 6.78 5.13
25 6.94 5.26 8.61 6.41
26 4.73 3.58 4.83 3.65
27 4.73 3.58 4.83 3.65
28 6.94 5.26 8.61 6.41

Calculation of Area of Steel

Span Position of Moments Moments (kNm) Mu/bd2 Pt % Ast in mm2 (reqd) Ast in mm2 (provided) Spacing of 10 mm bar @ c/c
Short Near Support 6.94 0.57 0.162 178.20 250 300
Mid Span 5.26 0.43 0.121 133.10 250 300
Long Near Support 8.61 0.71 0.204 224.40 250 300
Mid Span 6.41 0.53 0.150 165.00 250 300
Short Near Support 4.73 0.39 0.110 121.00 250 300
Mid Span 3.58 0.30 0.084 92.40 250 300
Long Near Support 4.83 0.40 0.113 124.30 250 300
Mid Span 3.65 0.30 0.084 92.40 250 300
Short Near Support 4.73 0.39 0.110 121.00 250 300
Mid Span 3.58 0.30 0.084 92.40 250 300
Long Near Support 4.83 0.40 0.113 124.30 250 300
Mid Span 3.65 0.30 0.084 92.40 250 300
Short Near Support 6.94 0.57 0.162 178.20 250 300
Mid Span 5.26 0.43 0.121 133.10 250 300
Long Near Support 8.61 0.71 0.204 224.40 250 300
Mid Span 6.41 0.53 0.150 165.00 250 300
Short Near Support 6.00 0.50 0.142 156.20 250 300
Mid Span 4.50 0.37 0.105 115.50 250 300
Long Near Support 6.78 0.56 0.159 174.90 250 300
Mid Span 5.13 0.42 0.119 130.90 250 300
Short Near Support 4.12 0.34 0.096 105.60 250 300
Mid Span 3.12 0.26 0.084 92.40 250 300
Long Near Support 4.17 0.34 0.096 105.60 250 300
Mid Span 3.13 0.26 0.084 92.40 250 300
Short Near Support 3.40 0.28 0.084 92.40 250 300
Mid Span 2.57 0.21 0.084 92.40 250 300
Long Near Support 4.00 0.33 0.093 102.30 250 300
Mid Span 3.00 0.25 0.084 92.40 250 300
Short Near Support 4.12 0.34 0.096 105.60 250 300
Mid Span 3.12 0.26 0.084 92.40 250 300
Long Near Support 4.17 0.34 0.096 105.60 250 300
Mid Span 3.13 0.26 0.084 92.40 250 300
Short Near Support 6.00 0.50 0.142 156.20 250 300
Mid Span 4.50 0.37 0.105 115.50 250 300
Long Near Support 6.78 0.56 0.145 159.50 250 300
Mid Span 5.13 0.42 0.119 130.90 250 300
Short Near Support 5.95 0.49 0.139 152.90 250 300
Mid Span 4.50 0.37 0.105 115.50 250 300
Long Near Support 6.78 0.56 0.159 174.90 250 300
Mid Span 5.13 0.42 0.119 130.90 250 300
Short Near Support 4.13 0.34 0.096 105.60 250 300
Mid Span 3.04 0.25 0.084 92.40 250 300
Long Near Support 4.17 0.34 0.096 105.60 250 300
Mid Span 3.13 0.26 0.084 92.40 250 300
Short Near Support 3.33 0.28 0.084 92.40 250 300
Mid Span 2.57 0.21 0.084 92.40 250 300
Long Near Support 3.89 0.32 0.090 99.00 250 300
Mid Span 2.92 0.24 0.084 92.40 250 300
Short Near Support 4.13 0.34 0.096 105.60 250 300
Mid Span 3.04 0.25 0.084 92.40 250 300
Long Near Support 4.17 0.34 0.096 105.60 250 300
Mid Span 3.13 0.26 0.084 92.40 250 300
Short Near Support 5.95 0.49 0.139 152.90 250 300
Mid Span 4.5 0.37 0.105 115.50 250 300
Long Near Support 6.78 0.56 0.159 174.90 250 300
Mid Span 5.13 0.42 0.119 130.90 250 300
Short Near Support 5.95 0.49 0.139 152.90 250 300
Mid Span 4.5 0.37 0.105 115.50 250 300
Long Near Support 6.78 0.56 0.159 174.90 250 300
Mid Span 5.13 0.42 0.119 130.90 250 300
Short Near Support 4.13 0.34 0.096 105.60 250 300
Mid Span 3.04 0.25 0.084 92.40 250 300
Long Near Support 4.17 0.34 0.096 105.60 250 300
Mid Span 3.13 0.26 0.084 92.40 250 300
Short Near Support 3.33 0.28 0.084 92.40 250 300
Mid Span 2.57 0.21 0.084 92.40 250 300
Long Near Support 3.89 0.32 0.090 99.00 250 300
Mid Span 2.92 0.24 0.084 92.40 250 300
Short Near Support 4.13 0.34 0.096 105.60 250 300
Mid Span 3.04 0.25 0.084 92.40 250 300
Long Near Support 4.17 0.34 0.096 105.60 250 300
Mid Span 3.13 0.26 0.084 92.40 250 300
Short Near Support 5.95 0.49 0.139 152.90 250 300
Mid Span 4.5 0.37 0.105 115.50 250 300
Long Near Support 6.78 0.56 0.159 174.90 250 300
Mid Span 5.13 0.42 0.119 130.90 250 300
Short Near Support 6 0.50 0.142 156.20 250 300
Mid Span 4.5 0.37 0.105 115.50 250 300
Long Near Support 6.78 0.56 0.159 174.90 250 300
Mid Span 5.13 0.42 0.119 130.90 250 300
Short Near Support 4.12 0.34 0.096 105.60 250 300
Mid Span 3.12 0.26 0.084 92.40 250 300
Long Near Support 4.17 0.34 0.096 105.60 250 300
Mid Span 3.13 0.26 0.084 92.40 250 300
Short Near Support 3.4 0.28 0.084 92.40 250 300
Mid Span 2.57 0.21 0.084 92.40 250 300
Long Near Support 4 0.33 0.093 102.30 250 300
Mid Span 3 0.25 0.084 92.40 250 300
Short Near Support 4.12 0.34 0.096 105.60 250 300
Mid Span 3.12 0.26 0.084 92.40 250 300
Long Near Support 4.17 0.34 0.096 105.60 250 300
Mid Span 3.13 0.26 0.084 92.40 250 300
Short Near Support 6 0.50 0.142 156.20 250 300
Mid Span 4.5 0.37 0.105 115.50 250 300
Long Near Support 6.78 0.56 0.159 174.90 250 300
Mid Span 5.13 0.42 0.119 130.90 250 300
Short Near Support 6.94 0.57 0.162 178.20 250 300
Mid Span 5.26 0.43 0.121 133.10 250 300
Long Near Support 8.61 0.71 0.204 224.40 250 300
Mid Span 6.41 0.53 0.150 165.00 250 300
Short Near Support 4.73 0.39 0.110 121.00 250 300
Mid Span 3.58 0.30 0.084 92.40 250 300
Long Near Support 4.83 0.40 0.113 124.30 250 300
Mid Span 3.65 0.30 0.084 92.40 250 300
Short Near Support 4.73 0.39 0.110 121.00 250 300
Mid Span 3.58 0.30 0.084 92.40 250 300
Long Near Support 4.83 0.40 0.113 124.30 250 300
Mid Span 3.65 0.30 0.084 92.40 250 300
Short Near Support 6.94 0.57 0.162 178.20 250 300
Mid Span 5.26 0.43 0.121 133.10 250 300
Long Near Support 8.61 0.71 0.204 224.40 250 300
Mid Span 6.41 0.53 0.150 165.00 250 300

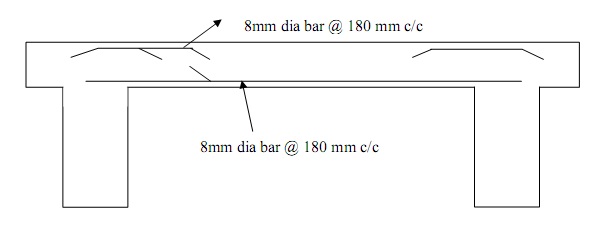
8mm dia

Panel Area of steel for maximum mid span moment (mm2) (3/4) Ast (mm2) 0.5 (3/4) Ast (mm2) Corner 1 Corner 2 Corner 3 Corner 4
1 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
2 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
3 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
4 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
5 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
6 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
7 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
8 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
9 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
10 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
11 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
12 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
13 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
14 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
15 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
16 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
17 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
18 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
19 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
20 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
21 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
22 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
23 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
24 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
25 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
26 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
27 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c
28 224.4 168.3 84.15 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c 8Φ@300 c/c

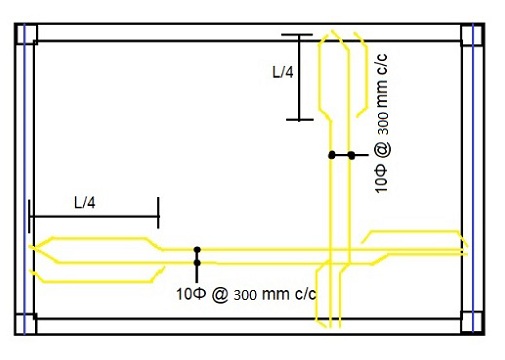
Slab Reinforcement Details
SLAB REINFORCEMENT DETAILS

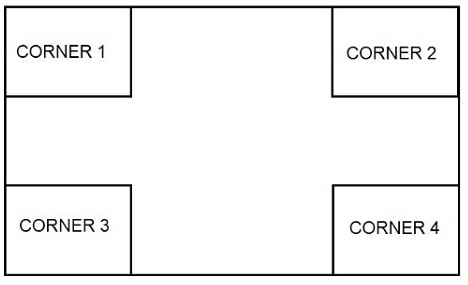
TORSIONAL REINFORCEMENT POSITION
TORSIONAL REINFORCEMENT POSITION

Share this post

Kanwarjot Singh

Kanwarjot Singh is the founder of Civil Engineering Portal, a leading civil engineering website which has been awarded as the best online publication by CIDC. He did his BE civil from Thapar University, Patiala and has been working on this website with his team of Civil Engineers.

If you have a query, you can ask a question here.

9 comments on "Design Calculation for Slab"

RITHIK says:
May 17, 2021 at 8:47 am

Mu/bd2 Pt % HOW YOU CALCULATED

Reply
Jyoti dhaygude says:
June 26, 2021 at 2:09 am

Design a cantilever slab of 1.5 m span to carry a super imposed load 2kN/m2 using m20 grade concrete and fe 415 grade steel?

Reply
Balabyekubo I. J. C says:
September 8, 2021 at 11:08 am

How to derive the formula Mu= 0.138fckbd2

Reply
Justice Chinedu says:
February 24, 2022 at 2:34 am

How did you get 15 in your cal for effective drpth

Reply
Vivek says:
July 26, 2022 at 1:38 pm

@RITHIK
Mu/bd2 Pt % HOW YOU CALCULATED

b=1000mm or 1 meter strip

=[6.94/(1000*110²)]*1000²
=0.57

Pt%
=AST/bd
=[178.20/(1000*110)]*100
=0.162%

Reply
Okike Joseph kenechukwu says:
September 1, 2022 at 6:27 am

That’s the concrete cover clearance

Reply
Eshetu Chali says:
December 26, 2022 at 4:55 pm

good

Reply
A.j.Emmanuel says:
January 2, 2023 at 5:12 pm

How to design 7mx7m slab r/d detail
The slab thickness 150mm r/f bar 10mm thickness 12 m bar grade of concrete g20
Density of concrete 2450kg/m3. I need video for r/f details.

Reply
Devendra Nikhade says:
March 17, 2024 at 9:35 pm

How to design slab and beam with live load of 15 Kn/m^2 for godown on First floor
Please reply asap

Reply

Leave a Reply

Your email address will not be published. Required fields are marked *

Journals Books & Softwares

Knowledge Center

Civil Engineering Branches

Ask a question